Polycons
Polycons have been devised by David Hirsch who patented the sphericon after having discoverd it (independently from others) in 1980. In comparison to other fields of Mathematics, polycons are hence relatively young.
The sphericon, which is dealt with in the article “polysphericons” is also a member of the family of polycons. It can be constructed as explained below with n=2, such that the sphericon can also be called (4,1)-polysphericon and 2-con.
Since the sphericon is a member of the polycon familiy, it does not surprise that polycons can roll. However, their rolling behaviour is very different from smooth rolling of a sphere (see paragraph and video below).
Constructing a polycon:
The procedure for a n-con is described below and can be understood easily with help of the illustrating pictures.
- Construct a cone with apex angle α = 180°-1/n·180°
- Cut off two wedges from this cone with angle β = 90° – 1/n·180°
- Repeat the steps above (n-1)-times and arrange them to form a base surface as 2n-gon
- Mirror this solid with respect to the x-y-plane
- Turn these two solids against each other by the angle of 1/n·180°
- The resulting n-con is sometimes also called according to the base surface. A 3-con can e.g. also be called hexacon.

Process to construct a n=3-con from left to right. The steps consist of cutting off two pieces of a half cone, duplicating it to form a solid with base surface 2n-gon (=hexagon) and mirroring it. 
One needs to turn the two halves against each other. 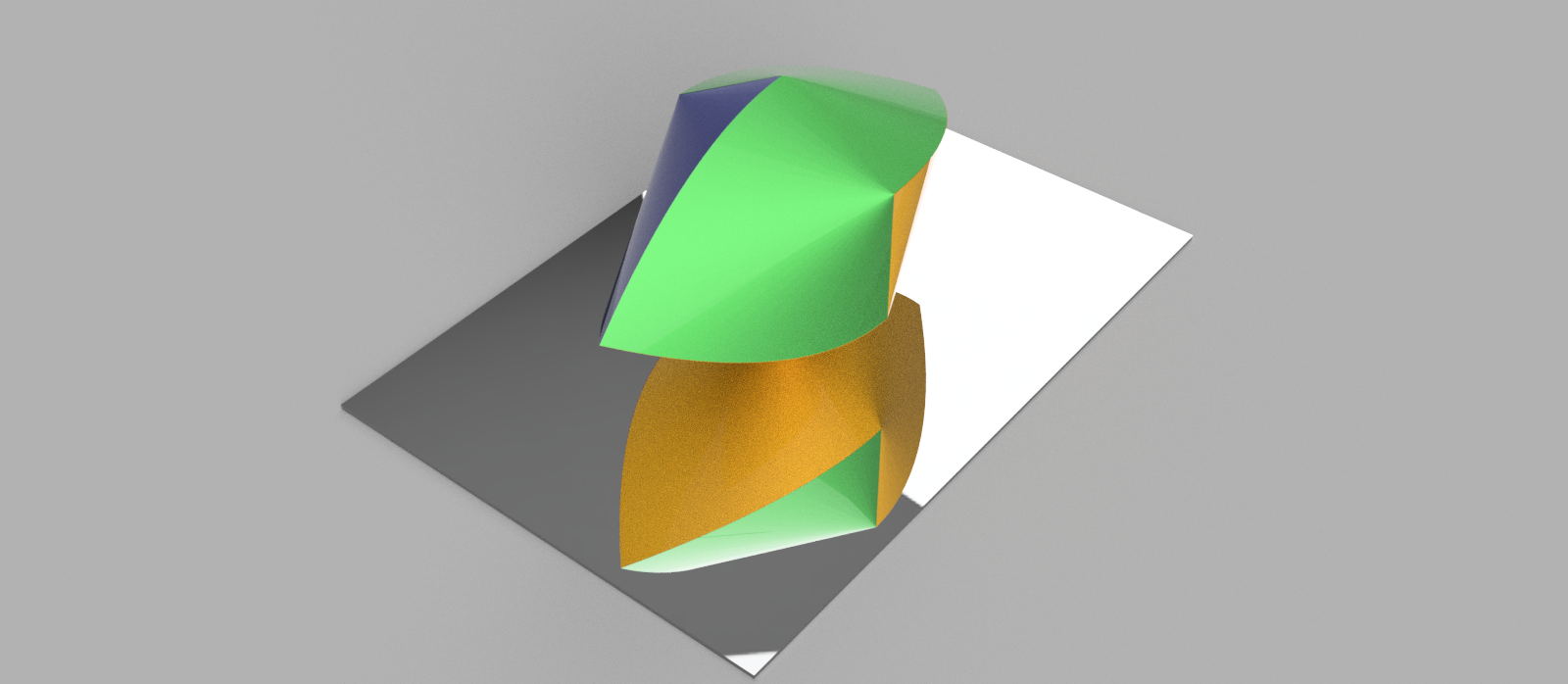
The resulting 3-con (=hexacon).
Rolling Polycons:
Polycones are developable rollers which means that all of the surface touches the ground while rolling. This is not the case for a cube and in particular for a sphere.
Unlike the oloid, the center of mass of a polycon is at constant height. One may now ask why the polycon does not move smoothly and why it seems to stumble. Due to symmetry, the center of mass must be in the middle of the polygon which is formed between the two halves. By watching the video, one can convince oneself that the center of mass wanders from left to right and vice versa. Seen from above, the trajectory of the center of mass consists of half circles.
Because the polycon is hence not moving in a straight line, it seems so roll clumsily.
Further information (links outside of HITS website):
- General information with addiotonal calculation for volume and surface area: https://arxiv.org/pdf/1901.10677.pdf
- Construction video of a 3-con : https://www.youtube.com/watch?v=tbH56N9mdX0